All Forum Posts by: Kim Hopkins

Quote from @Immanuel Sibero:

Quote from @Kim Hopkins:

Quote from @Immanuel Sibero:

Quote from @Kim Hopkins:

@Immanuel Sibero Awesome! Yes, there are several ways to prove it, but yours I think is the cleanest, great!

Now back to explaining this "in words", I love the direction you were going with that, but when you conclude: 

"
In this scenario, IF the debt holder demands 10% INTEREST can you see that you, as the owner, would also get 10% COC? The reason for this is because the property happens to be paying 10% CAP Rate, so everybody gets 10%!"

I believe you (of course, since we already proved it's true), but I don't see why if the cap rate is 10% and the debt holder demands 10% in interest, then we get 10% in COC. Does that make sense to you?

@Kim Hopkins

I'm having trouble answering... lol, how about alternative answers below:

- Based on the formula I laid out, when cap rate is 10% and interest rate is 10% then by definition COC is 10%.

- As owner, your portion of the property's rate of return is residual (whatever is left). Lender's portion of the property's rate of return is contractual (first dib). If the lender's contractual rate of return is higher than the property's rate of return, then the owner's rate of return would be lower than the property's rate of return... and vice versa. By the same logic, if the lender's contractual rate of return is the same as the the property's rate of return, then the owner's rate of return would necessarily have to be the same as the property's rate of return.

- If cap rate is 10% and debt holder demands 10% then COC can NOT be higher than 10% because this would require cap rate to be higher than 10%. In the same way, COC can NOT be lower than 10% because this would require cap rate to be lower than 10%. So COC has no choice but be 10%!

Which of the above do you like? :-)

Cheers... Immanuel

 Hi @Immanuel Sibero! I've been working on applications of this concept all week. I have some good stuff to share but I need to iron out the kinks first. 

In terms of your option of explanations "in words"... I'm going to add one to the mix... 

It's really just a general rule about ratios and proportionality: 

If A/B = C/D then (A-C)/(B-D) = A/B = C/D. 

In words: 

if A is proportional to B, and C is equally proportional to D   then the difference of A and B is equally proportional to the difference of C and D.

So it's really just about understanding WHY this general statement is true. 

I tried to discuss it with ChatGPT, but I swear that guy is not as smart as everyone says he is...I still don't intuitively see it. 

But nonetheless, the application is if the cap rate (NOI/PP) is equal to the mortgage constant (DS/LA), then the differences are equally proportional, and that's the cash on cash (NOI-DS)/(PP-LA) by definition.

By the way, the same is true for a regular loan, not just interest only! 

Now I'm trying to get a list of relationships like this between the cap rate and interest rate that effect the cash on cash, so that I can easily analyze any property using these simple inputs .... stay tuned ... 

But nonetheless, the application is if the cap rate (NOI/PP) is equal to the mortgage constant (DS/LA), then the differences are equally proportional, and that's the cash on cash (NOI-DS)/(PP-LA) by definition.

I was not a math major, maybe that's why I'm having trouble answering your question. But I feel like I'm going in circle. As normally defined, COC = CF/DP where CF is Cashflow and DP is down payment. I can restate your statement above with substitutions in bold font... and it would also be true:

But nonetheless, the application is if the cap rate (NOI/PP) is equal to the COC (CF/DP), then the differences are equally proportional, and that's the Interest (NOI-CF)/(PP-DP) by definition.

Are you NOT interested in the “why” for this proportional equality?


By the way, the same is true for a regular loan, not just interest only!

Interest Only has been a necessary assumption in all my responses, so I don't agree with this statement. If you were to amortize the loan by 1 dollar, COC would go out of synch (i.e. no longer equal to interest rate or cap rate). But since you made the claim, you have the burden of proof... lol. So how would you show that with an amortizing loan, when i = C, then COC also = C?? I just don't see it possible.


Last comment for today... here's another quote from your earlier post:
I've written it all out with actual math proofs for myself. I can't believe this doesn't exist anywhere. Can't find it anywhere online. It's incredibly helpful for analyzing an existing portfolio or a new acquisition since you can very quickly understand what adding debt to a property will or will not do to it's COC.

So, property analysis seems to be your bottom line (i.e. not necessarily the math connections between debt and COC). Well I made a post quite sometime ago that covers exactly this, that is, the impact of debt terms on COC... So YES, it does exist and it's been written before :-) Here is the excerpt:

I was evaluating a property with the following metrics:
Cap Rate: 6%
Interest Rate: 4%
LTV: 75%
Since the spread between cap rate and interest rate was slim (2%), I knew COC would be low. Since low COC means running the risk of negative cash flow which means risk of not paying the loan, I wanted to know how the various loan terms affect COC. For example, how sensitive was COC to the Cap and Int spread. This can easily be done using sensitivity tables in Excel. Based on the financial data of the property, following are two sensitivity tables:

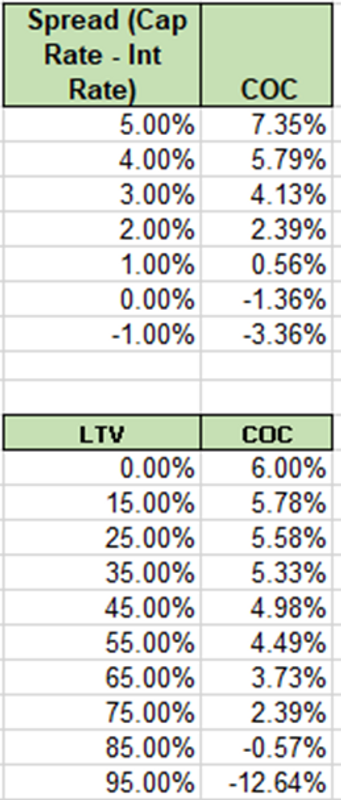
As you can see, my Cap rate - Int rate spread is 2% and from the first table it puts my COC at 2.39% which is concerning. Note that this table shows how sensitive COC is against the spread.

Second table shows how sensitive COC is against LTV. It shows that 75% debt is about the most I should borrow. Anything higher can put me in negative cashflow. HTH

Cheers... Immanuel

 Hi @Immanuel Sibero

I'm writing this from my phone so I can definitely clarify more later from the computer if needed, but this is getting exciting so I wanted to respond now!

First, I am very interested into WHY the proportionality holds in the interest only example. That's why I keep asking you to say it 10 different ways, but none of them have exactly clicked yet for me :-)

Now moving on to the example of a normal loan that is not interest only.

In this case, there is something called the mortgage constant M, AKA mortgage capitalization rate, which is defined as the debt service divided by the loan amount, DS/LA. By definition M*LA = DS. If you look at the formula for this,

M = i/(1-(1+i/12)^(-12Y) where i is the interest rate, and Y is the amortization years. You can see clearly that this number is independent of the amount of the loan LA, or the LTV.

Fix an amortization rate Y. And write the notation for M as M(i) to indicate that it is a function of the interest rate i.

Then here is the analog for a regular loan of what we have been discussing for an interest only loan:

Suppose you have a property with cap rate C.

Then there is one unique interest rate, I, so that the mortgage capitalization rate M(I) equals the cap rate C. 

M(I) = C.

Then for any interest rates i with i< I, your cash on cash will increase as a function of loan amount. In other words the more loan you take out, the better your cash on cash.

Conversely, for any interest rate i bigger than I, your cash on cash will decrease as a function of the loan amount. So in particular, your cap rate for that property is going to be bigger than any cash on cash return with any amount of debt. In other words, the property will perform better without debt than with debt, regardless of the loan amount.

So in the example you did with the sensitivity analysis, you could just calculate I, and then you know instantly that any interest rates less than I will give you cash on cash better than your cap rate, and any interest rates greater than I will give you cash on cash less than your cap rate and will decrease with the more loan you take out. 

 Hi @Immanuel Sibero! I've been working on applications of this concept all week. I have some good stuff to share but I need to iron out the kinks first. 

In terms of your option of explanations "in words"... I'm going to add one to the mix... 

It's really just a general rule about ratios and proportionality: 

If A/B = C/D then (A-C)/(B-D) = A/B = C/D. 

In words: 

if A is proportional to B, and C is equally proportional to D   then the difference of A and B is equally proportional to the difference of C and D.

So it's really just about understanding WHY this general statement is true. 

I tried to discuss it with ChatGPT, but I swear that guy is not as smart as everyone says he is...I still don't intuitively see it. 

But nonetheless, the application is if the cap rate (NOI/PP) is equal to the mortgage constant (DS/LA), then the differences are equally proportional, and that's the cash on cash (NOI-DS)/(PP-LA) by definition.

By the way, the same is true for a regular loan, not just interest only! 

Now I'm trying to get a list of relationships like this between the cap rate and interest rate that effect the cash on cash, so that I can easily analyze any property using these simple inputs .... stay tuned ... 

Someone on my team figured it out! This is called the mortgage constant or the mortgage capitalization rate. More to come... there's a lot this little guy tells you about a deal...

Here's more info on it, in case anyone finds this thread...

https://www.investopedia.com/terms/m/mortgageconstant.asp

@Immanuel Sibero Awesome! Yes, there are several ways to prove it, but yours I think is the cleanest, great!

Now back to explaining this "in words", I love the direction you were going with that, but when you conclude: 

"
In this scenario, IF the debt holder demands 10% INTEREST can you see that you, as the owner, would also get 10% COC? The reason for this is because the property happens to be paying 10% CAP Rate, so everybody gets 10%!"

I believe you (of course, since we already proved it's true), but I don't see why if the cap rate is 10% and the debt holder demands 10% in interest, then we get 10% in COC. Does that make sense to you?

Hi @Immanuel Sibero and @David M. , I'm excited for more math brains to discuss this with! Let's understand this very simple example first: 

Suppose it's an interest only loan. Define the variables: interest rate i, annual debt service DS, loan amount LA, purchase price PP, net operating income NOI, cash on cash COC, and cap rate C.

Since it's an interest only loan, 

i x LA = DS (interest rate times loan amount equals annual debt service).

Now suppose the interest rate equals the cap rate: i = C.

Then I claim the COC = i = C. In other words, the COC is equal to both the interest rate and cap rate.

Here's the proof: 

Since i = C, DS/LA = NOI/PP.

That's the same as saying NOI/DS = PP/LA. Call this ratio r.

So r = NOI/DS = PP/LA.

Then NOI - DS = r*DS - DS = (r-1) * DS

and PP - LA = r*LA - LA = (r-1)*LA.

So COC = (NOI - DS)/(PP-LA) (by definition)

which is = (r-1)*DS/(r-1)*LA = DS/LA = i = C. 

I'm trying to understand this in WORDS though. If the rate of payment (interest rate i) equals the rate of return (cap rate C), then WHY is the return on investment (COC) also equal to these rates? I can understand it mathematically, but not in practice.

@Immanuel Siberoundefined

 Good point. I shouldn't call it the "effective interest rate". That name is already taken. It should be called the "effective payment rate" or something like that because it gives you the total annual debt payment (including principal). This isn't the same as the effective annual interest rate you mentioned above which is only giving you interest. 

I have a math question for you! I'm trying to analyze our portfolio and have gotten into some pretty deep math calculations regarding interest rates and debt payments.

I want to know: is there a name for the percentage, call it "R", that is your total annual loan payment P divided by your total loan amount PV? 

I would call it the "effective interest rate" or something like that because R x PV = P. (The effective interest rate times your loan amount equals your annual loan payment).

To say it another way, in the classic loan amount formula below, is there a name for the "rate" R := r/(1-(1+r)^(-n)) circled below in red?

 Hey @David M., wow did this get me in a rabbit hole! I figured it out. I think I need to explain it in a fresh thread because of my variety of typos above, but here's the basic idea.

It is not actually true that the COC (Cash on Cash) is monotonically decreasing with increasing debt.

The simplest example is an interest only loan.

If you have an interest only loan, and your interest rate is less than the cap rate, then COC is INREASING as a function of debt. In other words, the more debt you take on, the higher your COC.

If your interest rate is higher than your cap rate, then COC is DECREASING as a function of debt. In other words, the more debt you take on, the worse your COC gets.

Lastly, if your interest rate is equal to your cap rate, then your COC is actually equal to your interest rate and your cap rate.

A similar phenomenon is true if it's not an interest only loan, but it's more complicated. 

I've written it all out with actual math proofs for myself. I can't believe this doesn't exist anywhere. Can't find it anywhere online. It's incredibly helpful for analyzing an existing portfolio or a new acquisition since you can very quickly understand what adding debt to a property will or will not do to it's COC.

After over a decade of doing this, I still don't understand how the debt terms directly affect the Cash on Cash return of a deal. 

For simplicity, define CAP Rate (:= NOI/Purchase Price) and COC (:= Cash Flow/Total Cash Invested).

Assume Cash Flow := NOI - Debt Service, and Purchase Price := Total Cash Invested + Debt Service.

• Say you are considering buying Property A. If you buy Property A WITHOUT debt, then CAP Rate = COC because Debt Service = $0 so Cash Flow = NOI and Purchase Price = Total Cash Invested.

• If you choose to add debt to Property A, then Total Cash Invested goes down (since you're using debt), so the denominator of COC goes down, so COC could potentially go up. HOWEVER, Cash Flow also goes down, because now you have to pay Debt Service, so the numerator of COC also goes down, so your COC could potentially go down as well.

The "math" question is what is an easy way to determine if the COC is going to go up or down, based on the terms of the debt?

For example, if the interest rate for the debt is less than the CAP rate, does that mean the COC will always go up? I don't think so. I think it depends on the LTV and possibly other factors.

Is there any simple relationship here? 

 @Allan Smith Hi, sorry for the delay. I'm back to this project now and still stuck! 

You said you were calculating ROE as: 

Profit / (FMV - Loan Balance).

Agree with the denominator. It's the numerator I'm questioning. 

Do you define Profit as: 

Profit := NOI - One-Time-Expenses (Capex, Lease Commissions etc) - Debt Service?

If you're comparing ROE of an existing asset to COC of a new asset, then Profit should definitely be after debt service, since that's accounted for in the definition of COC.

But for one-time expenses, if you are only looking at the ROE of an existing asset for one year (say the prior year) and you put on a new roof, you don't want to conclude the property has a terrible ROE from this one time expense. 

That's why I'm saying "Profit" in ROE should be defined as: 

Profit := NOI - Debt Service.

But this definition doesn't exist anywhere. So...still confused.